spinQuest-Preis für junge Wissenschaftler für Stefan Schulte
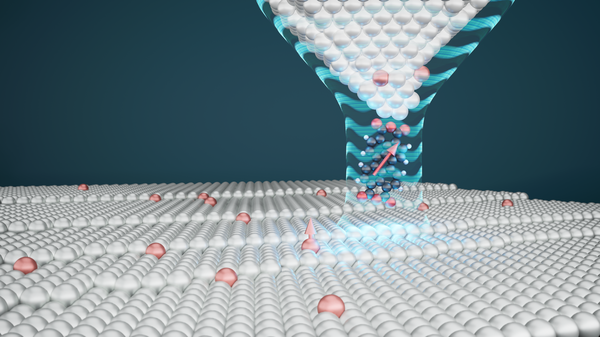
„Kann ein molekularer Quantenpunkt auf der STM-Spitze die Oberflächenpolarisierbarkeit abbilden?“ Für die Beantwortung dieser Frage wurde Stefan Schulte, Doktorand am PGI-3, auf der Konferenz „spin based quantum science and technology (spinQueST) 2025“ in Ascona, Schweiz, mit dem spinQueST-Preis für junge Wissenschaftler ausgezeichnet. In seinem Poster zeigt er, wie der resonante Transport durch eine mit einem organischen Molekül (NTCDA) funktionalisierte STM-Spitze Orbitalstrukturen und die Geometrie der Spitze offenbart und dass die Elektron-Phonon-Kopplung die Empfindlichkeit des mit Molekülen funktionalisierten STM erhöht, wodurch letztlich eine Polarisierbarkeitskarte der NTCDA-Schichten auf der Probe entsteht, die „dunkle“ Moleküle mit einer höheren Ladungsdichteverteilung verbindet. Übersetzt mit DeepL.com (kostenlose Version)